Technical Library TEMPERAMENTS XXV: Bach/O’Donnell Entire Contents Copyright © 2011 CBH

Dr Lehman’s Bach Temperament attracted controversy in the musical world as soon as it was published in February 2005. The squiggle squabble continues. There is more than one way to skin a cat, we say in English, and so perhaps there can indeed be more than one “Bach Temperament”.

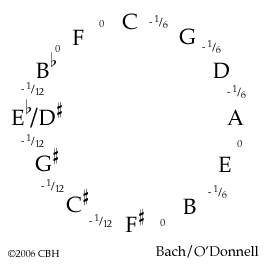
In the November 2006 issue of Early Music, under the title “Bach’s temperament, Occam’s razor, and the Neidhardt factor” Australian musicologist, organist and harpsichordist Dr John O’Donnell discussed the several problems he found with the Bach/Lehman temperament, and then proposed his own version. The abstract of Dr O’Donnell’s article can be viewed on the Oxford University Press website.

While his possible solution is again based on the squiggle found on the title page of Das wohltemperirte Clavier, it is regarded linearly in its original orientation. Indeed, any student knows the preludes and fugues of WTC are arranged chromatically, not by the circle of fifths.

Another major divergence from Bach/Lehman is Dr O’Donnell’s interpretation of the single and double loops not as the strict size of tempered fifths, but rather as during the tuning process using widened 4ths and narrowed 5ths respectively.

If you’re familiar with Vallotti, you will know the sound of a sixth-comma fifth well enough, and won’t have any particular trouble with this temperament. There are a few more tuning steps, and it’s a little more complicated but not impossible. Unlike Vallotti, here we have only four pure fifths, with four fifths narrowed by a sixth-comma, and the remaining four tempered half as much to make them in fact the same size as equal-tempered fifths. Piano tuners and beat counters will be happier if I observe that any sixth-comma fifth must beat twice as fast an equal-tempered fifth on the same notes, eg at A440, d'–a' must theoretically beat at 1.8 times per second. (That’s close enough to two beats per second for me.)

It is entirely possible to follow the diagram above and work around the whole circle in fifths and fourths as proposed in the article, tuning each fifth the appropriate size straight off. But here’s one practical method of putting everything in order and tuning the Bach/O’Donnell temperament from scratch:

1. Tune your a' to a tuning fork, and tune a in absolute perfect tune a beatless octave below it.

2. Now we want to set the f a third below that a: Tune it pure first of all, but then widen the interval by flattening the f until you hear three distinct beats per second. This is only a temporary position for the f—we must eventually flatten it some more.

3. Using the temporary position of F, we are going to narrow all the fifths in the circle between F and A by exactly the same amount, a sixth-comma. All these tempered fifths are narrow, so must be squeezed. Tune middle c' pure to f, then squeeze the interval by lowering your middle c' a little until you hear a lazy beat of a bit more than once a second.

4. Tune d' pure to a', then raise your d' a little, again squeezing the interval so it has a perceptible but not overly blatant wave. As I remarked in our preliminaries above, this interval will beat a tad less than twice per second, so check it against the second hand on your watch until you have a good wow-wow, wow-wow. It helps to repeat the interval every second or so—listen when the notes are fresh so you don’t have to struggle to hear the beats while the sound is dying away.

5. Tune g a beatless fifth below d', then squeeze the interval by raising the g. Compare f–c' to g–d': These two intervals are the same size, so they should sound pretty similar. The f–c' fifth is a tone lower, so if your intervals are to be the same theoretical size, f–c' should beat marginally slower than g–d', but don’t fuss needlessly.

6. Tune g' up an octave from g. Compare c'–g' to d'–a': These two intervals are the same size, so again they should sound pretty similar. If not, a little error may have crept in, so juggle all these fifths until you are happy with their uniform roughness. Don’t move your a, nor your f just yet!

7. So far, our results are identical to Vallotti, but now we diverge. Refer to the diagram, and see what we must do next on the sharp side—a quick and pure fifth that the violinists will love, because they won’t have to flatten their top string: Tune your e' a pure fifth above a, and drop down an octave to e.

8. You now require a narrow fifth between e and b, and you know enough to check the e–b to f–c' and g–d' to ensure all those intervals are indeed the same sixth-comma narrow, perhaps with the perceptible beats accelerating slightly as you ascend.

9. Tune a pure fifth from b up to f♯', and drop down a pure octave to f♯.

10. Let’s skip to the flat side of C now: The two pure fifths there should be easy enough. Retune your f below middle c', lowering it to make a perfectly beatless interval. This is where f belongs. Tune up an octave from f to f' and down another pure fifth to b♭.

11. With only three notes remaining to be determined, we are almost done. The four fifths which occur together in the circle between F♯ and B♭ are narrow and in fact equal-tempered in size—barely out of tune. If you’ve got this far, you are enough of a magician to squeeze them almost imperceptibly, cramming them into our circle to keep Pythagoras’ ghost at rest. As if you need me to tell you, determine your c♯' a pure fifth above f♯ and squeeze the interval only slightly this time by lowering your c♯'. Drop down a pure octave to c♯, and up a pure fifth to g♯ before squeezing the c♯–g♯ slightly by lowering the top note.

12. Lastly, find your dual-purpose e♭/ d ♯ a pure fifth below b♭ then squeezing it ever so slightly by raising the e♭. Tune up an octave to e♭' and see what sort of fifth remains to your already tuned g♯. With any luck, no adjustment is necessary because the fifth g♯–d♯' (e♭') is already slightly narrow.

If you’re wondering why I’m so particular about getting you to tune all your intervals pure before manipulating them, it’s simply because tempered intervals beat whether they are wide or narrow, and we want to be sure that in this temperament, all the tempered fifths are indeed narrow. Make a final check, if you like, verifying that your fifths are the same size as the diagram shows.

Bring the rest of the instrument in tune with your bearings area. Now you are ready to play some music again, and see if Bach/O’Donnell suits you.

Further discussion
Anonymous [Kayano, Moxzan] Dodecagon — Chi-s akt temo Tokyo 2012, p95

	Pitch nomenclature
	Harpsichord Tuning Process
	Tuning Bibliography
	Technical Library overview
	Harpsichords Australia Home Page